Chapter 1 – Similarity
Theorems to study for the Chapter
Practice set 1.1
1. Base of a triangle is 9 and height is 5. Base of another triangle is 10 and height is 6. Find the ratio of areas of these triangles.

Given:
Let the area, base and height of the first triangle be A\(_1\), b\(_1\) and h\(_1\) respectively.
Let the area, base and height of the second triangle be A\(_2\), b\(_2\) and h\(_2\) respectively.
∴ b\(_1\) = 9
h\(_1\) = 5
b\(_2\) = 10
h\(_2\) = 6
To Find:
Ratio of areas of the two triangles.
Solution:
\(\large \frac {A_1}{A_2}\) = \(\large \frac {b_1\, × \,h_1}{b_2\, ×\, h_2}\) … [The ratio of areas of two triangles is equal to the ratio of the product of their bases and corresponding heights]
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {9 \,× \,5}{10\,× \,6}\) …[Given]
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {3}{4}\)
Ans: The ratio of the areas of the triangles is 3 : 4
2. In figure 1.13 BC ⊥ AB, AD ⊥ AB, BC = 4, AD = 8, then find \(\large \frac {A (△ABC)}{A (△ADB)}\).
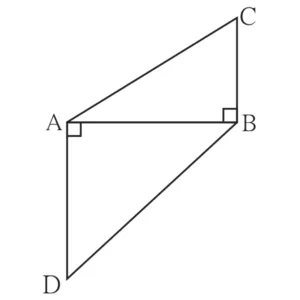
Given:
BC ⊥ AB
AD ⊥ AB
BC = 4
AD = 8
To Find:
\(\large \frac {A (△ABC)}{A (△ADB)}\)
Solution:
In △ABC and △ADB,
Side AB is the common base
∴ \(\large \frac {A (△ABC)}{A (△ADB)}\) = \(\large \frac {BC}{AD}\) … [The ratio of areas of two triangles having equal bases, is equal to the ratio of their corresponding heights]
∴ \(\large \frac {A (△ABC)}{A (△ADB)}\) = \(\large \frac {4}{8}\) …[Given]
∴ \(\large \frac {A (△ABC)}{A (△ADB)}\) = \(\large \frac {1}{2}\)
Ans: \(\large \frac {A (△ABC)}{A (△ADB)}\) = \(\large \frac {1}{2}\)
3. In adjoining figure 1.14, seg PS ⊥ seg RQ, seg QT ⊥ seg PR. If RQ = 6, PS = 6 and PR = 12, then find QT.
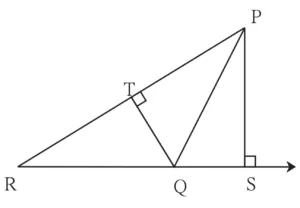
Given:
seg PS ⊥ seg RQ
seg QT ⊥ seg PR
RQ = 6
PS = 6
PR = 12
To Find:
QT
Solution:
Area of a triangle = \(\large \frac {1}{2}\) × base × height
∴ A (∆PQR) = \(\large \frac {1}{2}\) × RQ × PS
∴ A (∆PQR) = \(\large \frac {1}{2}\) × 6 × 6
∴ A (∆PQR) = 18 sq. units
Also,
A (∆PQR) = \(\large \frac {1}{2}\) × PR × QT
∴ 18 = \(\large \frac {1}{2}\) × 12 × QT
∴ QT = \(\large \frac {18\,×\,2}{12}\)
∴ QT = 3 units
Ans: QT = 3 units
4. In adjoining figure, AP ⊥ BC, AD || BC, then find A (△ABC) : A (△BCD).
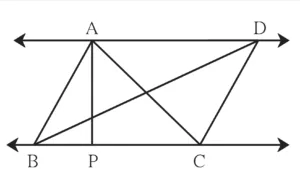
Given:
AP ⊥ BC
AD || BC
To Find:
A (△ABC) : A (△BCD)
Solution:
In △ABC and △BCD,
∴ \(\large \frac {A (△ABC)}{A (△BCD)}\) = \(\large \frac {AP\,× \,BC}{AP\,× \,BC}\)
∴ \(\large \frac {A (△ABC)}{A (△BCD)}\) = 1
Ans: \(\large \frac {A (△ABC)}{A (△BCD)}\) = 1
5. In adjoining figure PQ ⊥ BC, AD ⊥ BC then find following ratios.
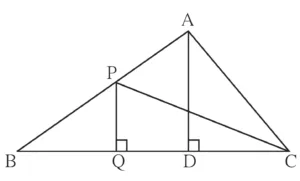
(i) \(\large \frac {A (△PQB)}{A (△PBC)}\)
Solution:
In ∆PQB and ∆PBC,
PQ is the common height
∴ \(\large \frac {A (∆PQB)}{A (∆PBC)}\) = \(\large \frac {BQ}{BC}\) … [The ratio of areas of two triangles having equal heights, is equal to the ratio of their corresponding bases]
Ans: \(\large \frac {A (∆PQB)}{A (∆PBC)}\) = \(\large \frac {BQ}{BC}\)
(ii) \(\large \frac {A (△PBC)}{A (△ABC)}\)
Solution:
In ∆PBC and ∆ABC,
BC is the common base
∴ \(\large \frac {A (∆PBC)}{A (∆ABC)}\) = \(\large \frac {PQ}{AD}\) … [The ratio of areas of two triangles having equal bases, is equal to the ratio of their corresponding heights]
Ans: \(\large \frac {A (∆PBC)}{A (∆ABC)}\) = \(\large \frac {PQ}{AD}\)
(iii) \(\large \frac {A (△ABC)}{A (△ADC)}\)
Solution:
In ∆ABC and ∆ADC,
AD is the common height
∴ \(\large \frac {A (∆ABC)}{A (∆ADC)}\) = \(\large \frac {BC}{DC}\) … [The ratio of areas of two triangles having equal heights, is equal to the ratio of their corresponding bases]
Ans: \(\large \frac {A (∆ABC)}{A (∆ADC)}\) = \(\large \frac {BC}{DC}\)
(iv) \(\large \frac {A (△ADC)}{A (△PQC)}\)
Solution:
In ∆ADC and ∆PQC,
\(\large \frac {A (∆ADC)}{A (∆PQC)}\) = \(\large \frac {DC\,× \,AD}{QC\,× \,PQ}\)
Ans: \(\large \frac {A (∆ADC)}{A (∆PQC)}\) = \(\large \frac {DC\,× \,AD}{QC\,× \,PQ}\)
Practice set 1.2
1. Given below are some triangles and lengths of line segments. Identify in which figures, ray PM is the bisector of ∠QPR.
(i)
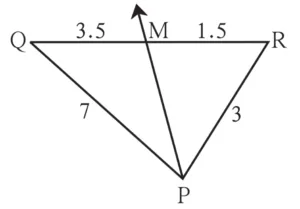
Solution:
In ∆PQR,
\(\large \frac {PQ}{PR}\) = \(\large \frac {7}{3}\) …(i)
\(\large \frac {QM}{RM}\) = \(\large \frac {3.5}{1.5}\)
\(\large \frac {QM}{RM}\) = \(\large \frac {7}{3}\) …(ii)
∴ \(\large \frac {PQ}{PR}\) = \(\large \frac {QM}{RM}\) …[From (i) and (ii)]
∴ Ray PM bisects ∠QPR
(ii)
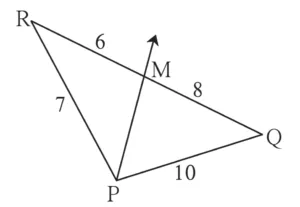
Solution:
In ∆PQR,
\(\large \frac {PQ}{PR}\) = \(\large \frac {10}{7}\) …(i)
\(\large \frac {QM}{RM}\) = \(\large \frac {8}{6}\)
\(\large \frac {QM}{RM}\) = \(\large \frac {4}{3}\) …(ii)
∴ \(\large \frac {PQ}{PR}\) ≠ \(\large \frac {QM}{RM}\) …[From (i) and (ii)]
∴ Ray PM does not bisect ∠QPR
(iii)
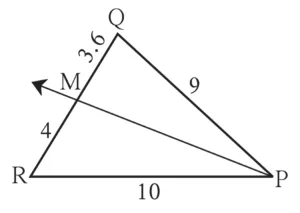
In ∆PQR,
\(\large \frac {PQ}{PR}\) = \(\large \frac {9}{10}\) …(i)
\(\large \frac {QM}{RM}\) = \(\large \frac {3.6}{4}\)
\(\large \frac {QM}{RM}\) = \(\large \frac {9}{10}\) …(ii)
∴ \(\large \frac {PQ}{PR}\) = \(\large \frac {QM}{RM}\) …[From (i) and (ii)]
∴ Ray PM bisects ∠QPR
2. In ∆PQR, PM = 15, PQ = 25, PR = 20, NR = 8. State whether line NM is parallel to side RQ. Give a reason.
Given:
In ∆PQR,
PM = 15
PQ = 25
PR = 20
NR = 8
To find:
Whether line NM is parallel to side RQ with reason.
Solution:
In ∆PQR,
PQ = PM + MQ …[P – M – Q]
∴ 25 = 15 + MQ
∴ MQ = 25 – 15
∴ MQ = 10
Also,
PR = PN + NR …[P – N – R]
∴ 20 = PN + 8
∴ PN = 20 – 8
∴ PN = 12
Now,
\(\large \frac {PM}{MQ}\) = \(\large \frac {15}{10}\)
∴ \(\large \frac {PM}{MQ}\) = \(\large \frac {3}{2}\)
and \(\large \frac {PN}{NR}\) = \(\large \frac {12}{8}\)
∴ \(\large \frac {PN}{NR}\) = \(\large \frac {3}{2}\) …(ii)
∴ \(\large \frac {PM}{MQ}\) = \(\large \frac {PN}{NR}\) …[From (i) and (ii)]
∴ line NM ∥ side QR …[By Converse of Basic Proportionality Theorem]
Ans: Line NM is parallel to side QR.
3. In △MNP, NQ is a bisector of ∠N. If MN = 5, PN = 7 MQ = 2.5 then find QP.
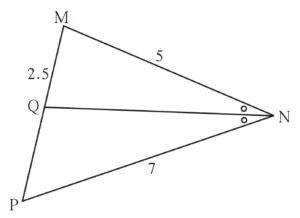
Given:
In △MNP
NQ is a bisector of ∠N
MN = 5
PN = 7
MQ = 2.5
To find:
QP
Solution:
In ∆MNP,
NQ bisects ∠MNP …[Given]
∴ \(\large \frac {MN}{PN}\) = \(\large \frac {MQ}{QP}\) …[By Angle bisector property of a triangle]
∴ \(\large \frac {5}{7}\) = \(\large \frac {2.5}{QP}\)
∴ 5 × QP = 2.5 × 7
∴ QP = \(\large \frac {2.5\, × \,7}{5}\)
∴ QP = 3.5 units
Ans: The value of QP is 3.5 units.
4. Measures of some angles in the figure are given. Prove that \(\large \frac {AP}{PB}\) = \(\large \frac {AQ}{QC}\)
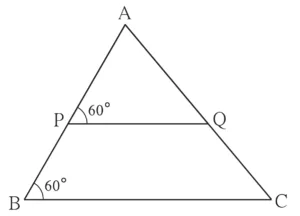
Given:
∠APQ = 60⁰
∠ABC = 60⁰
To Prove:
\(\large \frac {AP}{PB}\) = \(\large \frac {AQ}{QC}\)
Proof:
In ∆ABC,
∠APQ ≅ ∠ABC …[Given]
∴ seg PQ ∥ side BC …(i) [By Corresponding angles test]
∴ \(\large \frac {AP}{PB}\) = \(\large \frac {AQ}{QC}\) …[By Basic Proportionality Theorem]
Hence proved that \(\large \frac {AP}{PB}\) = \(\large \frac {AQ}{QC}\)
5. In trapezium ABCD, side AB || side PQ || side DC, AP = 15, PD = 12, QC = 14, find BQ.

Given:
In trapezium ABCD,
side AB || side PQ || side DC
AP = 15
PD = 12
QC = 14
To find:
PQ
Solution:
In trapezium ABCD,
side AB || side PQ || side DC …[Given]
∴ \(\large \frac {AP}{PD}\) = \(\large \frac {BQ}{QC}\) …[By the Property of three parallel lines and their transversal]
∴ \(\large \frac {15}{12}\) = \(\large \frac {BQ}{14}\)
∴ BQ = \(\large \frac {15 \,× \,14}{12}\)
∴ BQ = 17.5 units
Ans: The value of BQ is 17.5 units.
6. Find QP using given information in the figure.
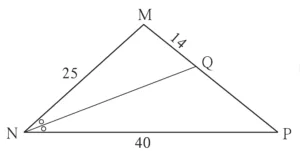
Given:
MN = 25
MQ = 14
NP = 40
To find:
QP
Solution:
In ∆MNP,
NQ bisects ∠MNP …[Given]
∴ \(\large \frac {MN}{PN}\) = \(\large \frac {MQ}{QP}\) …[By Angle bisector property of a triangle]
∴ \(\large \frac {25}{40}\) = \(\large \frac {14}{QP}\)
∴ 25 × QP = 14 × 40
∴ QP = \(\large \frac {14\, × \,40}{25}\)
∴ QP = 22.4 units
Ans: The value of QP is 22.4 units.
7. In figure 1.41, if AB || CD || FE then find x and AE.
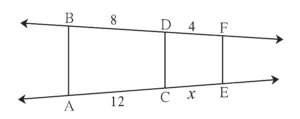
Given:
AB || CD || FE
BD = 8
DF = 4
AC = 12
CE = x
To find:
The value of x and AE
Solution:
seg AB || seg CD || seg EF …[Given]
∴ \(\large \frac {AC}{CE}\) = \(\large \frac {BD}{DF}\) …[By the Property of three parallel lines and their transversals]
∴ \(\large \frac {12}{x}\) = \(\large \frac {8}{4}\)
∴ x = \(\large \frac {12\, × \,4}{8}\)
∴ x = 6 units
Now,
AE = AC + CE …[A – C – E]
AE = 12 + 6 …[Given]
∴ AE = 18 units
Ans: The value of x is 6 units and AE is 18 units.
8. In △LMN, ray MT bisects ∠LMN. If LM = 6, MN = 10, TN = 8, then find LT.
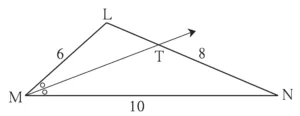
Given:
In △LMN,
Ray MT bisects ∠LMN
LM = 6
MN = 10
TN = 8
To find:
LT
Solution:
In ∆LMN,
Ray MT bisects ∠LMN …[Given]
∴ \(\large \frac {LM}{MN}\) = \(\large \frac {LT}{TN}\) …[By Angle bisector property of a triangle]
∴ \(\large \frac {6}{10}\) = \(\large \frac {LT}{8}\)
∴ 6 × 8 = LT × 10
∴ LT = \(\large \frac {6\, × \,8}{10}\)
∴ LT = 4.8 units
Ans: The value of QP is 4.8 units.
9. In △ABC, seg BD bisects ∠ABC. If AB = x, BC = x + 5, AD = x – 2, DC = x + 2, then find the value of x.
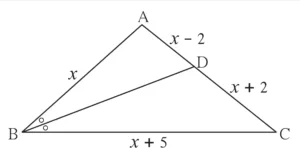
Given:
In △ABC,
Seg BD bisects ∠ABC
AB = x
BC = x + 5
AD = x – 2
DC = x + 2
To find:
The value of x
Solution:
In ∆ABC,
Ray BD bisects ∠ABC …[Given]
∴ \(\large \frac {AB}{BC}\) = \(\large \frac {AD}{DC}\) …[By Angle bisector property of a triangle]
∴ \(\large \frac {x}{x\,+\,5}\) = \(\large \frac {x\,–\,2}{x\,+\,2}\)
∴ x (x + 2) = (x + 5) (x – 2)
∴ x² + 2x = x² + 5x – 2x – 10
∴ x² + 2x = x² + 3x – 10
∴ x² + 2x – x² – 3x = –10
∴ – x = – 10
∴ x = 10
∴ x = 10
Ans: The value of x is 10.
10. In the figure 1.44, X is any point in the interior of the triangle. Point X is joined to vertices of the triangle. Seg PQ || seg DE, seg QR || seg EF. Fill in the blanks to prove that, seg PR || seg DF.
Proof :
In △XDE, PQ || DE … ________
∴ \(\large \frac {XP}{▢}\) = \(\large \frac {▢}{QE}\) …. (I) (Basic proportionality theorem)
In △XEF, QR || EF … ________
∴ \(\large \frac {▢}{▢}\) = \(\large \frac {▢}{▢}\) …(II) ________
∴ \(\large \frac {▢}{▢}\) = \(\large \frac {▢}{▢}\) …from (I) and (II)
∴ seg PR || seg DE … (Converse of basic proportionality theorem)
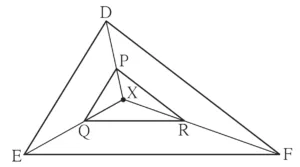
Solution:
In△XDE, PQ || DE …[Given]
∴ \(\large \frac {XP}{PD}\) = \(\large \frac {SQ}{QE}\) …. (i) [By Basic proportionality theorem]
In△XEF, QR || EF …[Given]
∴ \(\large \frac {XQ}{QE}\) = \(\large \frac {XR}{RF}\) …(ii) [By Basic proportionality theorem]
∴ \(\large \frac {XP}{PD}\) = \(\large \frac {XR}{RD}\) …from (i) and (ii)
∴ seg PR || seg DE …[By Converse of basic proportionality theorem]
11. In △ABC, ray BD bisects ∠ABC and ray CE bisects ∠ACB. If seg AB ≅ seg AC then prove that ED || BC.
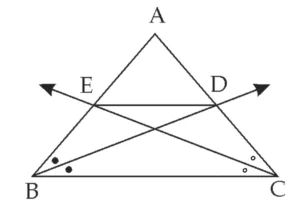
Given:
In△ABC,
Ray BD bisects ∠ABC
Ray CE bisects ∠ACB
seg AB ≅ seg AC
To Prove:
ED || BC
Proof:
In ∆ABC,
Ray BD bisects ∠ABC …[Given]
∴ \(\large \frac {AB}{BC}\) = \(\large \frac {AD}{DC}\) …(i) [By Angle bisector property of a triangle]
Ray CE bisects ∠ACB …[Given]
∴ \(\large \frac {AC}{BC}\) = \(\large \frac {AE}{BE}\) …(ii) [By Angle bisector property of a triangle]
seg AB ≅ seg AC …(iii) [Given]
∴ \(\large \frac {AB}{BC}\) = \(\large \frac {AE}{BE}\) …(iv) [From (ii) and (iv)]
∴ In ∆ABC,
\(\large \frac {AD}{DC}\) = \(\large \frac {AE}{BE}\) …[From (i) and (iv)]
∴ seg ED || side BC …[By Converse of Basic proportionality theorem]
Hence proved that seg ED || side BC
Practice set 1.3
1. In figure 1.55, ∠ABC = 75°, ∠EDC = 75° state which two triangles are similar and by which test? Also write the similarity of these two triangles by a proper one to one correspondence.
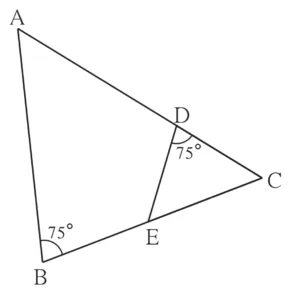
Given:
∠ABC = 75°
∠EDC = 75°
To Find:
Whether the two triangles are similar and by which test
One to one correspondence of the two triangles
Solution:
In ∆ABC and ∆EDC
∠ABC ≅ ∠EDC …[Each angle is 75⁰]
∠ECD ≅ ∠ACB …[Common angle]
∴ ∆ABC ~ ∆EDC …[By AA test for similarity of triangles]
One to One Correspondence,
ABC ↔ EDC
Ans: ∆ABC ~ ∆EDC by AA test for similarity of triangles and ABC ↔ EDC is one to one correspondence.
2. Are the triangles in figure 1.56 similar? If yes, by which test?
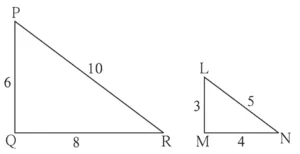
Given:
PQ = 6
QR = 8
PR = 10
LM = 3
MN = 4
LN = 5
To Find:
Whether the triangles are similar.
Solution:
In ∆PQR and ∆LMN,
\(\large \frac {PQ}{LM}\) = \(\large \frac {6}{3}\)
∴ \(\large \frac {PQ}{LM}\) = \(\large \frac {2}{1}\) …(i)
\(\large \frac {QR}{MN}\) = \(\large \frac {8}{4}\)
∴ \(\large \frac {QR}{MN}\) = \(\large \frac {2}{1}\) …(ii)
\(\large \frac {PR}{LN}\) = \(\large \frac {10}{5}\)
∴ \(\large \frac {PR}{LN}\) = \(\large \frac {2}{1}\) …(iii)
\(\large \frac {PQ}{LM}\) = \(\large \frac {QR}{MN}\) = \(\large \frac {PR}{LN}\) …[From (i), (ii) and (iii)]
∴ ∆PQR ~ ∆LMN …[By SSS test of similarity of triangles]
Ans: The triangles are similar.
3. As shown in figure 1.57, two poles of height 8 m and 4 m are perpendicular to the ground. If the length of shadow of a smaller pole due to sunlight is 6 m then how long will the shadow of the bigger pole be at the same time?
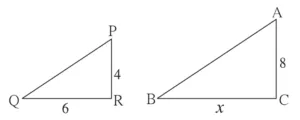
Given:
PR = 4 m
AC = 8 m
QR = 6 m
To Find:
BC
Solution:
In ∆PQR and ∆ABC,
∠PRQ ≅ ∠ACB …[Both are 90⁰]
∠QPR ≅ ∠BAC …[Angles made by the sunlight from the top are congruent]
∆PQR ~ ∆ABC …[By AA test of similarity of triangles]
∴ \(\large \frac {PR}{AC}\) = \(\large \frac {QR}{BC}\) …[Corresponding sides are proportional]
∴ \(\large \frac {4}{8}\) = \(\large \frac {6}{BC}\) …[Given]
∴ BC = \(\large \frac {8\, × \,6}{4}\)
∴ BC = 12 m
Ans: Length of the shadow casted by the longer pole is 12 m.
4. In ∆ABC, AP ⊥ BC, BQ ⊥ AC, B – P – C, A – Q – C then prove that, ∆CPA ~ ∆CQB. If AP = 7, BQ = 8, BC = 12 then find AC.
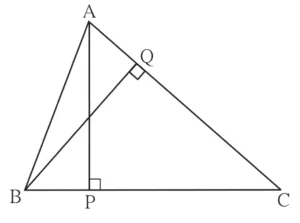
Given:
AP ⊥ BC
BQ ⊥ AC
B – P – C
A – Q – C
AP = 7
BQ = 8
BC = 12
To Prove:
∆CPA ~ ∆CQB
To Find:
AC
Proof:
In ∆CPA and ∆CQB,
∠ACP ≅ ∠BCQ …[Common angle]
∠APC ≅ ∠BQC …[Each is 90⁰]
∴ ∆CPA ~ ∆CQB …[By AA test for similarity] … (i)
Solution:
∵ ∆CPA ~ ∆CQB …[From (i)]
∴ \(\large \frac {AP}{BQ}\) = \(\large \frac {AC}{BC}\) …[Corresponding sides are proportional]
∴ \(\large \frac {7}{8}\) = \(\large \frac {AC}{12}\)
∴ AC = \(\large \frac {7\, ×\, 12}{8}\)
∴ AC = 10.5 units
Ans: ∆CPA ~ ∆CQB by AA test of similarity and the value of AC is 10.5 units.
5. Given : In trapezium PQRS, side PQ ∥ side SR, AR = 5AP, AS = 5AQ then prove that, SR = 5PQ
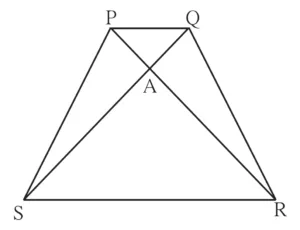
Given:
In trapezium PQRS,
side PQ ∥ side SR
AR = 5AP
AS = 5AQ
To Prove:
SR = 5PQ
Solution:
In ∆ASR and ∆AQP,
AR = 5AP …[Given]
∴ \(\large \frac {AR}{AP}\) = \(\large \frac {5}{1}\) …(i)
AS = 5AQ …[Given]
∴ \(\large \frac {AS}{AQ}\) = \(\large \frac {5}{1}\) …(ii)
∴ \(\large \frac {AR}{AP}\) = \(\large \frac {AS}{AQ}\) …[From (i) and (ii)]
∠SAR ≅ ∠QAP …[Vertically opposite angles]
∴ ∆ASR ~ ∆AQP …[By SAS Test of similarity of triangles]
∴ \(\large \frac {SR}{PQ}\) = \(\large \frac {AR}{AP}\) …[Corresponding sides are proportional]
∴ \(\large \frac {SR}{PQ}\) = \(\large \frac {5}{1}\) …[From (i)]
∴ SR = 5 PQ
Hence proved.
6. In trapezium ABCD, (Figure 1.60) side AB ∥ side DC, diagonals AC and BD intersect in point O. If AB = 20, DC = 6, OB = 15 then find OD.

Given:
In trapezium ABCD,
side AB ∥ side DC,
Diagonals AC and BD intersect in point O
AB = 20
DC = 6
OB = 15
To Find:
OD
Solution:
In ∆AOB and ∆COD,
side AB ∥ side CD and AC is the transversal …[Given]
∠CAB ≅ ∠ACD …[Alternate angles theorem]
i.e. ∠OAB ≅ ∠OCD …[C – O – D, B – O – D]
∠AOB ≅ ∠COD …[Vertically opposite angles]
∴ ∆AOB ~ ∆COD …[By AA test for similarity of triangles]
∴ \(\large \frac {AB}{DC}\) = \(\large \frac {OB}{OD}\) …[Corresponding sides are proportional]
∴ \(\large \frac {20}{6}\) = \(\large \frac {15}{OD}\)
∴ OD = \(\large \frac {15\,×\,6}{20}\)
∴ OD = 4.5 units
Ans: The value of OD is 4.5 units.
7. □ ABCD is a parallelogram point E is on side BC. Line DE intersects ray AB in point T. Prove that DE × BE = CE × TE.
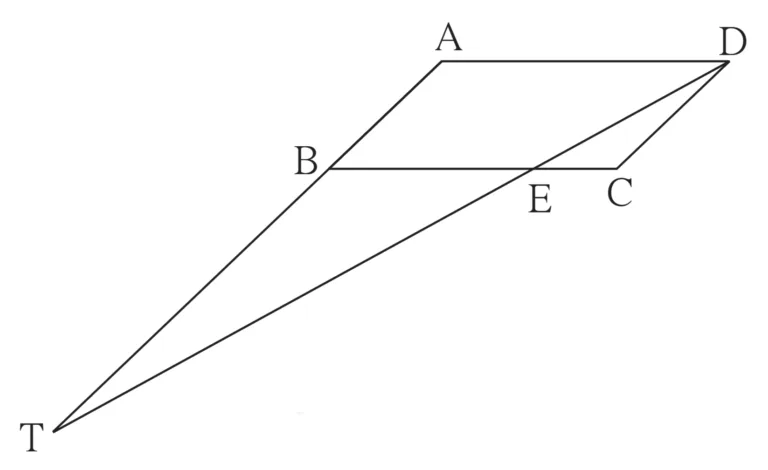
Given:
□ ABCD is a parallelogram
Point E is on side BC
Line DE intersects ray AB in point T
To Prove:
DE × BE = CE × TE
Proof:
□ ABCD is a parallelogram …[Given]
seg AB ∥ seg CD …[Opposite sides of a parallelogram are parallel]
seg AT ∥ seg CD …[A – B – T]
Now,
seg AT ∥ seg CD and TD is the transversal,
∴ ∠ATD ≅ ∠CDT …[Alternate angles theorem]
∴ ∠BTE ≅ ∠CDE …(i) [A – B – T, T – E – D]
In ∆BTE and ∆CDE,
∠BTE ≅ ∠CDE …[From (i)]
∠BET ≅ ∠CED …[Vertically opposite angles]
∴ ∆BTE ~ ∆CDE …[By AA test of similarity]
∴ \(\large \frac {BE}{CE}\) = \(\large \frac {TE}{DE}\) …[Corresponding sides are proportional]
∴ DE × BE = CE × TE
Hence proved.
8. In the figure, seg AC and seg BD intersect each other in point P and \(\large \frac {AP}{CP}\) = \(\large \frac {BP}{DP}\). Prove that, ∆ABP ~ ∆CDP.
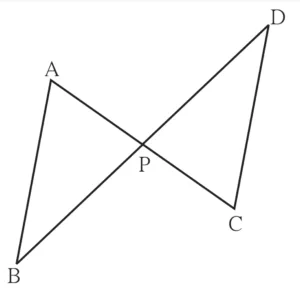
Given:
Seg AC and seg BD intersect each other in point P
\(\large \frac {AP}{CP}\) = \(\large \frac {BP}{DP}\)
To Prove:
∆ABP ~ ∆CDP
Proof:
In ∆ABP and ∆CDP,
\(\large \frac {AP}{CP}\) = \(\large \frac {BP}{DP}\) …[Given]
∠APB ≅ ∠CPD …[Vertically opposite angles]
∴ ∆ABP ~ ∆CDP …[SAS test of similarity of triangles]
Hence proved.
9. In the figure, in ∆ABC, point D on side BC is such that, ∠BAC = ∠ADC. Prove that, CA² = CB × CD
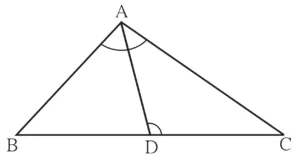
Given:
In ∆ABC,
∠BAC = ∠ADC
To Prove:
CA² = CB × CD
Proof:
In ∆ABC and ∆DAC,
∠BAC ≅ ∠ADC …[Given]
∠BCA ≅ ∠ACD …[Common Angle]
∴ ∆ABC ~ ∆DAC …[By AA test of similarity]
∴ \(\large \frac {CA}{CD}\) = \(\large \frac {CB}{CA}\) …[Corresponding sides are proportional]
∴ CA² = CB × CD
Hence proved.
Practice set 1.4
1. The ratio of corresponding sides of similar triangles is 3 : 5; then find the ratio of their areas.
Given:
Let the areas of two similar triangles be A\(_1\) and A\(_2\) and their corresponding sides S\(_1\) and S\(_2\) respectively.
∴ \(\large \frac {S_1}{S_2}\)
To Find:
\(\large \frac {A_1}{A_2}\)
Solution:
\(\large \frac {S_1}{S_2}\) …[Given]
Both the triangles are similar …[Given]
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {(S_1)²}{(S_2)²}\) …[Theorem on areas of similar triangles]
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {3²}{5²}\)
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {9}{25}\)
Ans: The ratio of the areas of two similar triangles is 9 : 25.
2. If ∆ABC ~ ∆PQR and AB: PQ = 2:3, then fill in the blanks.
\(\large \frac {A(∆ABC)}{A(∆PQR)}\) = \(\large \frac {AB²}{□}\) = \(\large \frac {2²}{3²}\) = \(\large \frac {□}{□}\)
Solution:
\(\large \frac {A(∆ABC)}{A(∆PQR)}\) = \(\large \frac {AB²}{PQ²}\) = \(\large \frac {2²}{3²}\) = \(\large \frac {4}{9}\)
3. If ∆ABC ~ ∆PQR, A(∆ABC) = 80, A(∆PQR) = 125, then fill in the blanks.
\(\large \frac {A(∆ABC)}{A(∆□)}\) = \(\large \frac {80}{125}\)
∴ \(\large \frac {AB}{PQ}\) = \(\large \frac {□}{□}\)
Solution:
\(\large \frac {A(∆ABC)}{A(∆PQR)}\) = \(\large \frac {80}{125}\)
∴ \(\large \frac {AB}{PQ}\) = \(\large \frac {4}{5}\)
4. ∆LMN ~ ∆PQR, 9 × A (∆PQR) = 16 × A (∆LMN). If QR = 20 then find MN.
Given:
∆LMN ~ ∆PQR
9 × A (∆PQR) = 16 × A (∆LMN)
QR = 20
To Find:
MN
Solution:
In ∆LMN and ∆PQR,
9 × A(∆PQR) = 16 × A (∆LMN) …[Given]
∴ \(\large \frac {9}{16}\) = \(\large \frac {A(∆LMN)}{A(∆PQR)}\)
i.e. \(\large \frac {A(∆LMN)}{A(∆PQR)}\) = \(\large \frac {9}{16}\) …(i)
\(\large \frac {A(∆LMN)}{A(∆PQR)}\) = \(\large \frac {MN²}{QR²}\) …[Theorem on areas of similar triangles]
∴ \(\large \frac {9}{16}\) = \(\large \frac {MN²}{20²}\)
∴ \(\large \sqrt{\frac {9}{16}}\) = \(\large \sqrt{\frac {MN²}{20²}}\) …[By taking square root]
∴ \(\large \frac {3}{4}\) = \(\large \frac {MN}{20}\)
∴ MN = \(\large \frac {3 \,×\, 20}{4}\)
∴ MN = 15
Ans: MN = 15 units
5. Areas of two similar triangles are 225 sq.cm. and 81 sq.cm. If a side of the smaller triangle is 12 cm, then find the corresponding side of the bigger triangle.
Given:
Let the areas of two similar triangles be A\(_1\) and A\(_2\) and their corresponding sides S\(_1\) and S\(_2\) respectively.
∴ A\(_1\) = 225 cm²
A\(_2\) = 81 cm²
S\(_2\) = 12 cm
Triangles are similar
To Find:
S\(_1\)
Solution:
Since both the triangles are similar …[Given]
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {(S_1)²}{(S_2)²}\) …[Theorem on areas of similar triangles]
∴ \(\large \frac {225}{81}\) = \(\large \frac {S_1²}{12²}\)
∴ \(\large \sqrt{\frac {225}{81}}\) = \(\large \sqrt{\frac {(S_1)²}{12²}}\) …[By taking square root]
∴ \(\large \frac {15}{9}\) = \(\large \frac {S_1}{12}\)
S\(_1\) = \(\large \frac {15\,×\, 12}{9}\)
∴ S\(_1\) = 20 cm
Ans: The corresponding side of the bigger triangle is 20 cm.
6. ∆ABC and ∆DEF are equilateral triangles. If A(∆ABC) : A (∆DEF) = 1 : 2 and AB = 4, find DE.
Given:
∆ABC and ∆DEF are equilateral triangles
A(∆ABC) : A (∆DEF) = 1 : 2
AB = 4
To Find:
DE
Solution:
∆ABC and ∆DEF are equilateral triangles.
We know that equilateral triangles are congruent, hence they are similar
∴ ∆ABC ~ ∆DEF
∴ \(\large \frac {A(∆ABC)}{A(∆DEF}\) = \(\large \frac {AB²}{DE²}\) …[Theorem on areas of similar triangles]
∴ \(\large \frac {1}{2}\) = \(\large \frac ({4²}{DE²})\)
∴ \(\large \sqrt{\frac {1}{2}}\) = \(\large \sqrt{\frac {4²}{DE²}}\) …[By taking square root]
∴ \(\large \frac {1}{sqrt{9}}\) = \(\large \frac {4}{DE}\)
∴ DE = 4 \(\large \sqrt{2}\)
Ans: The value of DE is 4 \(\large \sqrt{2}\)
7. In figure 1.66, seg PQ || seg DE, A(∆PQF) = 20 units, PF = 2 DP, then find A(□DPQE) by completing the following activity.
A(∆PQF) = 20 units, PF = 2 DP,
Let us assume DP = x.
∴ PF = 2x
DF = DP + □ = □ + □ = 3x
In ∆FDE and ∆FPQ,
∠FDE ≅ ∠□ … corresponding angles
∠FED ≅ ∠□ … corresponding angles
∴ ∆FDE ~ ∆FPQ … AA test
∴ \(\large \frac {A(∆FDE)}{A(∆FPQ)}\) = \(\large \frac {□}{□}\) = \(\large \frac {(3x)²}{(2x)²}\) = \(\large \frac {9}{4}\)
A(∆FDE) = \(\large \frac {9}{4}\) × A(∆FPQ) = \(\large \frac {9}{4}\) × □ = □
A(□DPQE) = A(∆FDE) – A(∆FPQ)
A(□DPQE) = □ – □
A(□DPQE) = □

Solution:
A(∆PQF) = 20 units, PF = 2 DP,
Let us assume DP = x.
∴ PF = 2x
DF = DP + PF = x + 2x = 3x
In ∆FDE and ∆FPQ,
∠FDE ≅ ∠FPQ … corresponding angles
∠FED ≅ ∠FQP … corresponding angles
∴ ∆FDE ~ ∆FPQ … AA test
∴ \(\large \frac {A(∆FDE)}{A(∆FPQ)}\) = \(\large \frac {DF²}{PF²}\) = \(\large \frac {(3x)²}{(2x)²}\) = \(\large \frac {9}{4}\)
A(∆FDE) = \(\large \frac {9}{4}\) × A(∆FPQ) = \(\large \frac {9}{4}\) × 20 = 45
A(□DPQE) = A(∆FDE) – A(∆FPQ)
A(□DPQE) = 45 – 20
A(□DPQE) = 25 sq. units.
Problem Set 1
1. Select the appropriate alternative.
(1) In ∆ABC and ∆PQR, in a one to one correspondence \(\large \frac {AB}{QR}\) = \(\large \frac {BC}{PR}\) = \(\large \frac {CA}{PQ}\) then
(A) ∆PQR ~ ∆ABC
(B) ∆PQR ~ ∆CAB
(C) ∆CBA ~ ∆PQR
(D) ∆BCA ~ ∆PQR

Ans: Option (B) – ∆PQR ~ ∆CAB
Explanation:
AB ↔ QR
BC ↔ PR
CA ↔ PQ
∴ ∆ABC ~ ∆QRP or ∆CAB ~ ∆PQR
(2) If in ∆DEF and ∆PQR, ∠D ≅ ∠Q, ∠R ≅ ∠E, then which of the following statements is false?
(A) \(\large \frac {EF}{PR}\) = \(\large \frac {DF}{PQ}\)
(B) \(\large \frac {DE}{PQ}\) = \(\large \frac {EF}{RP}\)
(C) \(\large \frac {DE}{QR}\) = \(\large \frac {DF}{PQ}\)
(D) \(\large \frac {EF}{RP}\) = \(\large \frac {DE}{QR}\)
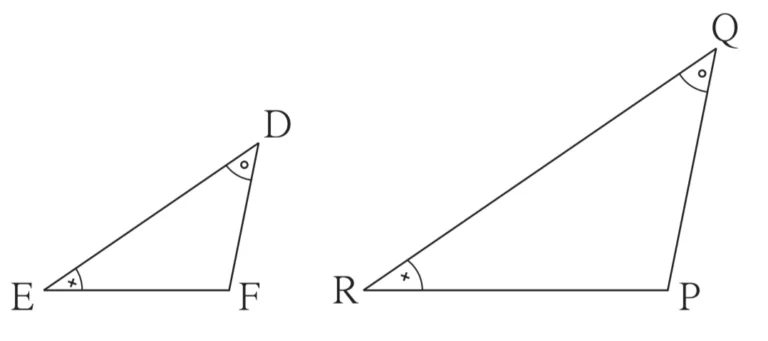
Ans: Option (B) – \(\large \frac {DE}{PQ}\) = \(\large \frac {EF}{RP}\)
Explanation:
In ∆DEF and ∆PQR,
∠D ≅ ∠Q …[Given]
∠R ≅ ∠E …[Given]
∴ ∆DEF ~ ∆PQR …[By AA test of similarity of triangles]
∴ \(\large \frac {EF}{PR}\) = \(\large \frac {DF}{PQ}\) = \(\large \frac {DE}{QR}\) …[Corresponding sides are proportional]
(3) In ∆ABC and ∆DEF ∠B = ∠E, ∠F = ∠C and AB = 3DE then which of the statements regarding are the two triangles is true?
(A) The triangles are not congruent and not similar
(B) The triangles are similar but not congruent.
(C) The triangles are congruent and similar.
(D) None of the statements above is true.
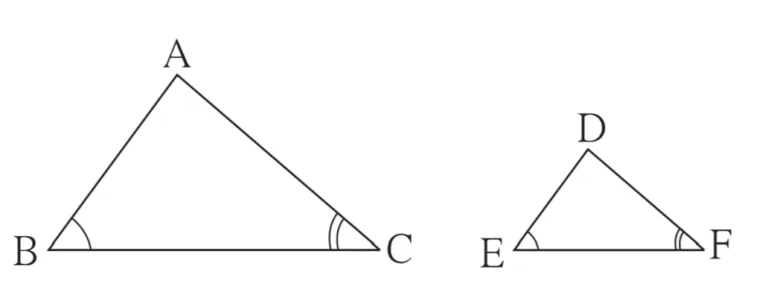
Ans: Option (B) – The triangles are similar but not congruent.
Explanation:
In ∆ABC and ∆DEF,
∠B ≅ ∠E …[Given]
∠F ≅ ∠C …[Given]
∴ ∆ABC ~ ∆DEF …[By AA test of similarity of triangles]
And,
AB = 3DE …[Given]
Which means ∆DEF must be three times larger than ∆ABC
∴ ∆ABC ~ ∆DEF but ∆ABC ≇ ∆DEF
(4) ∆ABC and ∆DEF are equilateral triangles, A (∆ABC) : A(∆DEF) = 1 : 2. If AB = 4 then what is the length of DE?
(A) 2 \(\sqrt{2}\)
(B) 4
(C) 8
(D) 4 \(\sqrt{2}\)
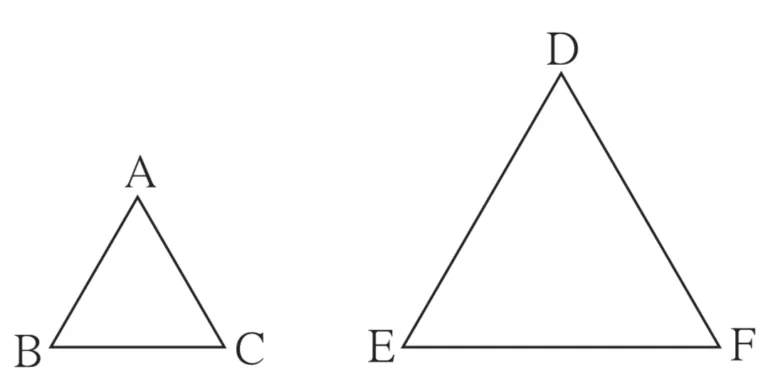
Ans: Option (D) – 4 \(\sqrt{2}\)
Explanation:
∆ABC and ∆DEF are equilateral triangles …[Given]
In ∆ABC and ∆DEF,
∠B ≅ ∠E
∠A ≅ ∠D …[Each angle of equilateral triangle is 60⁰]
∴ ∆ABC ~ ∆DEF …[By AA test of similarity of triangles]
∴ \(\large \frac {A(∆ABE)}{A(∆DEF)}\) = \(\large \frac {AB²}{DE²}\) …[Theorem on areas of similar triangles]
∴ \(\large \frac {1}{2}\) = \(\large \frac {4²}{DE²}\)
∴ \(\sqrt{\large \frac {1}{2}}\) = \(\sqrt{\large \frac {4²}{DE²}}\) …[By taking square root]
∴ \(\large \frac {1}{\sqrt{2}}\) = \(\large \frac {4}{DE}\)
∴ DE = 4 \(\sqrt{2}\) units
(5) In figure 1.71, seg XY || seg BC, then which of the following statements is true?
(A) \(\large \frac {AB}{AC}\) = \(\large \frac {AX}{AY}\)
(B) \(\large \frac {AX}{XB}\) = \(\large \frac {AY}{AC}\)
(C) \(\large \frac {AX}{YC}\) = \(\large \frac {AY}{XB}\)
(D) \(\large \frac {AB}{YC}\) = \(\large \frac {AC}{XB}\)
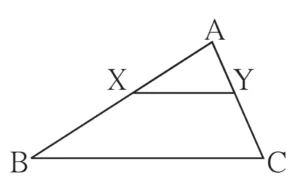
Ans: Option (A) – \(\large \frac {AB}{AC}\) = \(\large \frac {AX}{AY}\)
Explanation:
seg XY || seg BC,
∴ \(\large \frac {AX}{XB}\) = \(\large \frac {AY}{YC}\)
∴ \(\large \frac {XB}{AX}\) = \(\large \frac {YC}{AY}\) …[Invertendo]
∴ \(\large \frac {XB}{AX}\) + 1 = \(\large \frac {YC}{AY}\) + 1
∴ \(\large \frac {XB\,+ \,AX}{AX}\) = \(\large \frac {YC\, +\, AY}{AY}\)
∴ \(\large \frac {AB}{AX}\) = \(\large \frac {AC}{AY}\)
∴ \(\large \frac {AB}{AC}\) = \(\large \frac {AX}{AY}\) …[Alternendo]
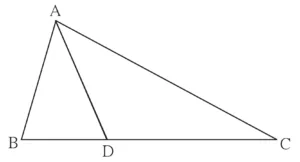
2. In ∆ABC, B – D – C and BD = 7, BC = 20 then find the following ratios.
(1) \(\large \frac {A(∆ABD) }{A(∆ADC)}\)
(2) \(\large \frac {A(∆ABD) }{A(∆ABC)}\)
(3) \(\large \frac {A(∆ADC) }{A(∆ABC)}\)
Given:
In ∆ABC,
B – D – C
BD = 7
BC = 20
To Find:
(1) \(\large \frac {A(∆ABD) }{A(∆ADC)}\)
(2) \(\large \frac {A(∆ABD) }{A(∆ABC)}\)
(3) \(\large \frac {A(∆ADC) }{A(∆ABC)}\)
Solution:
BC = BD + DC …(B – D – C)
∴ 20 = 7 + DC
∴ 20 – 7 = DC
∴ DC = 13 units.
∆ABD, ∆ADC and ∆ABC have a common vertex A and their bases BD, DC and BC lie on the same line BC.
∴ The heights of all three triangles are equal.
(1) \(\large \frac {A(∆ABD) }{A(∆ADC)}\) = \(\large \frac {BD}{DC}\) … [The ratio of areas of two triangles having equal heights, is equal to the ratio of their corresponding bases]
∴ \(\large \frac {A(∆ABD) }{A(∆ADC)}\) = \(\large \frac {7}{13}\)
(2) \(\large \frac {A(∆ABD) }{A(∆ABC)}\) = \(\large \frac {BD}{BC}\) … [The ratio of areas of two triangles having equal heights, is equal to the ratio of their corresponding bases]
∴ \(\large \frac {A(∆ABD) }{A(∆ABC)}\) = \(\large \frac {7}{20}\)
(3) \(\large \frac {A(∆ADC) }{A(∆ABC)}\) = \(\large \frac {DC}{BC}\) … [The ratio of areas of two triangles having equal heights, is equal to the ratio of their corresponding bases]
∴ \(\large \frac {A(∆ADC) }{A(∆ABC)}\) = \(\large \frac {13}{20}\)
Ans: The values are;
(1) \(\large \frac {A(∆ABD) }{A(∆ADC)}\) = \(\large \frac {7}{13}\)
(2) \(\large \frac {A(∆ABD) }{A(∆ABC)}\) = \(\large \frac {7}{20}\)
(3) \(\large \frac {A(∆ADC) }{A(∆ABC)}\) = \(\large \frac {13}{20}\)
3. Ratio of areas of two triangles with equal heights is 2 : 3. If the base of the smaller triangle is 6 cm then what is the corresponding base of the bigger triangle?
Given:
Let the area of the smaller triangle be A\(_1\) and its base be b\(_1\).
Let the area of the bigger triangle be A\(_2\) and its base be b\(_2\).
Triangles have equal heights.
\(\large \frac {A_1}{A_2}\) = \(\large \frac {2}{3}\)
b\(_1\) = 6 cm.
To Find:
b\(_2\)
Solution:
Triangles have equal heights …[Given]
∴ \(\large \frac {A_1}{A_2}\) = \(\large \frac {b_1}{b_2}\) …[The ratio of areas of two triangles having equal heights, is equal to the ratio of their corresponding bases]
∴ \(\large \frac {2}{3}\) = \(\large \frac {6}{b_2}\)
∴ b\(_2\) = \(\large \frac {3\,×\,6}{2}\)
∴ b\(_2\) = 9 cm
Ans: The base of the bigger triangle is 9 cm.
4. In figure 1.73, ∠ABC = ∠DCB = 90°, AB = 6, DC = 8 then \(\large \frac {A(∆ABC)}{A(∆DCB)}\) = ?
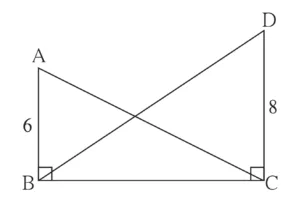
Given:
∠ABC = ∠DCB = 90°
AB = 6
DC = 8.
To Find:
\(\large \frac {A(∆ABC)}{A(∆DCB)}\)
Solution:
∆ABC and ∆DCB have a common base BC
∴ \(\large \frac {∆ABC}{∆DCB}\) = \(\large \frac {AB}{DC}\) …[The ratio of areas of two triangles having equal bases, is equal to the ratio of their corresponding heights]
∴ \(\large \frac {∆ABC}{∆DCB}\) = \(\large \frac {6}{8}\)
∴ \(\large \frac {∆ABC}{∆DCB}\) = \(\large \frac {3}{4}\)
Ans: \(\large \frac {∆ABC}{∆DCB}\) = \(\large \frac {3}{4}\)
5. In figure 1.74, PM = 10 cm, A(∆PQS) = 100 sq.cm, A(∆QRS) = 110 sq.cm, then find NR.
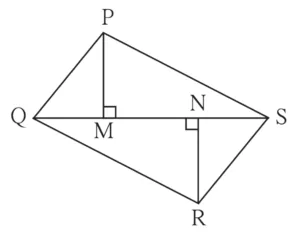
Given:
PM = 10 cm
A(∆PQS) = 100 sq.cm
A(∆QRS) = 110 sq.cm
To Find:
NR
Solution:
∆PQS and ∆QRS have a common base QS
∴ \(\large \frac {∆PQS}{∆QRS}\) = \(\large \frac {PM}{NR}\) …[The ratio of areas of two triangles having equal bases, is equal to the ratio of their corresponding heights]
∴ \(\large \frac {100}{110}\) = \(\large \frac {10}{NR}\)
∴ NR = \(\large \frac {10\,×\,110}{100}\)
∴ NR = 11 cm
Ans: The value of NR is 11 cm.
6. ∆MNT ~ ∆QRS. Length of altitude drawn from point T is 5 and length of altitude drawn from point S is 9. Find the ratio \(\large \frac {A(∆MNT)}{A(∆QRS)}\).
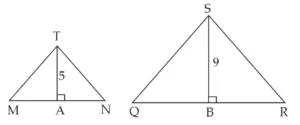
Given:
∆MNT ~ ∆QRS
Length of altitude drawn from point T is 5
Length of altitude drawn from point S is 9
To Find:
\(\large \frac {A(∆MNT)}{A(∆QRS)}\)
Solution:
∆MNT ~ ∆QRS …[Given]
∴ ∠M ≅ ∠Q …(i) [Corresponding angles are congruent]
In ∆MAT and ∆QBS
∠M ≅ ∠Q …[From (i)]
∠MAT ≅ ∠QBS …[Each is 90⁰]
∆MAT ~ ∆QBS …[By AA test of similarity of triangles]
\(\large \frac {TM}{SQ}\) = \(\large \frac {TA}{SB}\) …(ii) [Corresponding sides are proportional]
\(\large \frac {A(∆TMN)}{A(∆SQR)}\) = \(\large \frac {TM²}{SQ²}\) …[Theorem on areas of similar triangles]
\(\large \frac {A(∆TMN)}{A(∆SQR)}\) = \(\large \frac {TA²}{SQ²}\) …[From (ii)]
\(\large \frac {A(∆TMN)}{A(∆SQR)}\) = \(\large \frac {5²}{9²}\) …[Given]
\(\large \frac {A(∆TMN)}{A(∆SQR)}\) = \(\large \frac {25}{81}\)
Ans: \(\large \frac {A(∆TMN)}{A(∆SQR)}\) = \(\large \frac {25}{81}\)
7. In figure 1.75, A – D – C and B – E – C, seg DE || side AB If AD = 5, DC = 3, BC = 6.4 then find BE.
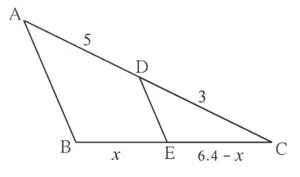
Given:
A – D – C and B – E – C
seg DE || side AB
AD = 5
DC = 3
BC = 6.4
To Find:
BE
Solution:
BC = BE + CE …[B – E – C]
∴ 6.4 = BE + CE
∴ CE = 6.4 – BE
In ∆ABC,
seg DE || side AB …[Given]
∴ \(\large \frac {AD}{DC}\) = \(\large \frac {BE}{EC}\) …[By Basic Proportionality theorem]
∴ \(\large \frac {5}{3}\) = \(\large \frac {x}{6.4\,-\,BE}\)
∴ 5 (6.4 – BE) = 3BE
∴ (6.4 × 5) – 5BE = 3BE
∴ 32 = 3BE + 5BE
∴ 32 = 8BE
∴ 32 = 8BE
∴ BE = \(\large \frac {32}{8}\)
∴ BE = 4 units
Ans: The value of BE is 4 units.
8. In the figure 1.76, seg PA, seg QB, seg RC and seg SD are perpendicular to line AD. AB = 60, BC = 70, CD = 80, PS = 280 then find PQ, QR and RS.

Given:
seg PA ⊥ line AD
seg QB ⊥ line AD
seg RC ⊥ line AD
seg SD ⊥ line AD
AB = 60
BC = 70
CD = 80
PS = 280
To Find:
PQ
QR
RS.
Solution:
seg PA ⊥ line AD
seg QB ⊥ line AD
seg RC ⊥ line AD
seg SD ⊥ line AD
∴ seg PA || seg QB || seg RC || seg SD …[If two or more lines are perpendicular to the same line then they are parallel to each other]
∴ PQ : QR : RS = AB : BC : CD …[By the Property of three parallel lines and their transversals]
∴ PQ : QR : RS = 60 : 70 : 80
∴ PQ : QR : RS = 6 : 7 : 8
Let the common multiple be x.
∴ PQ = 6x, QR = 7x, RS = 8x
∴ PS = PQ + QR + RS …[P – Q – R – S]
∴ 280 = 6x + 7x + 8x
∴ 21x = 280
∴ x = \(\large \frac {280}{21}\)
∴ x = \(\large \frac {40}{3}\)
∴ PQ = 6x = 6 × \(\large \frac {40}{3}\) = 80 units
QR = 7x = 7 × \(\large \frac {40}{3}\) = \(\large \frac {280}{3}\) units
RS = 8x = 8 × \(\large \frac {40}{3}\) = \(\large \frac {320}{3}\) units
Ans: The value of PQ is 80 units, QR is \(\large \frac {280}{3}\) units and RS is \(\large \frac {320}{3}\) units.
9. In ∆PQR seg PM is a median. Angle bisectors of ∠PMQ and ∠PMR intersect side PQ and side PR in points X and Y respectively. Prove that XY || QR. Complete the proof by filling in the boxes.
In ∆PMQ, ray MX is bisector of ∠PMQ.
∴ \(\large \frac {□}{□}\) = \(\large \frac {□}{□}\) ………. (I) theorem of angle bisector.
In ∆PMR, ray MY is bisector of ÐPMR.
∴ \(\large \frac {□}{□}\) = \(\large \frac {□}{□}\) ………. (II) theorem of angle bisector.
But \(\large \frac {MP}{MQ}\) = \(\large \frac {MP}{MR}\) ………. M is the midpoint QR, hence MQ = MR.
∴ \(\large \frac {PX}{XQ}\) = \(\large \frac {PY}{YR}\)
∴ XY || QR ………. converse of basic proportionality theorem.

Solution:
In ∆PMQ, ray MX is bisector of ∠PMQ.
∴ \(\large \frac {PM}{MQ}\) = \(\large \frac {PX}{XQ}\) ………. (I) theorem of angle bisector.
In ∆PMR, ray MY is bisector of ÐPMR.
∴ \(\large \frac {PM}{MR}\) = \(\large \frac {PY}{YR}\) ………. (II) theorem of angle bisector.
But \(\large \frac {MP}{MQ}\) = \(\large \frac {MP}{MR}\) ………. M is the midpoint QR, hence MQ = MR.
∴ \(\large \frac {PX}{XQ}\) = \(\large \frac {PY}{YR}\)
∴ XY || QR ………. converse of basic proportionality theorem.
10. In fig 1.78, bisectors of ∠B and ∠C of ∆ABC intersect each other in point X. Line AX intersects side BC in point Y. AB = 5, AC = 4, BC = 6 then find \(\large \frac {AX}{AY}\)
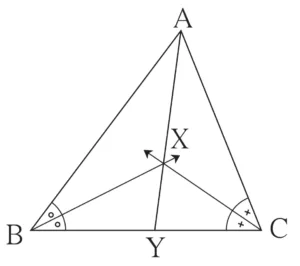
Given:
In ∆ABC,
Bisectors of ∠B and ∠C meet at point X
Line AX intersects side BC in point Y
AB = 5
AC = 4
BC = 6
To Find:
\(\large \frac {AX}{AY}\)
Solution:
In ∆ABY,
Ray BX bisects ∠ABY …[Given]
∴ \(\large \frac {AB}{BY}\) = \(\large \frac {AX}{XY}\) …(i) [Angle bisector property of a triangle]
In ∆ACY,
Ray CX bisects ∠ACY …[Given]
∴ \(\large \frac {AC}{CY}\) = \(\large \frac {AX}{XY}\) …(ii) [Angle bisector property of a triangle]
∴ \(\large \frac {AB}{BY}\) = \(\large \frac {AX}{XY}\) = \(\large \frac {AC}{CY}\) …[From (i) and (ii)]
∴ \(\large \frac {AB\,+\,AC}{BY\,+\,CY}\) = \(\large \frac {AX}{XY}\) …[By theorem on equal ratios]
∴ \(\large \frac {AB\,+\,AC}{BC}\) = \(\large \frac {AX}{XY}\) …[B – Y – C]
∴ \(\large \frac {5\,+\,4}{6}\) = \(\large \frac {AX}{XY}\)
∴ \(\large \frac {AX}{XY}\) = \(\large \frac {9}{6}\)
∴ \(\large \frac {AX}{XY}\) = \(\large \frac {3}{2}\)
Ans: \(\large \frac {AX}{XY}\) = \(\large \frac {3}{2}\)
11. In □ABCD, seg AD || seg BC. Diagonal AC and diagonal BD intersect each other in point P. Then show that \(\large \frac {AP}{PD}\) = \(\large \frac {PC}{BP}\)
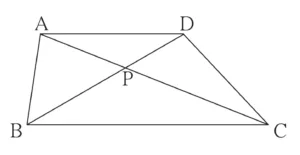
Given:
In □ABCD
seg AD || seg BC
Diagonal AC and diagonal BD intersect in point P
To Prove:
\(\large \frac {AP}{PD}\) = \(\large \frac {PC}{BP}\)
Solution:
In ∆APD and ∆CPB
seg AD || seg BC …[Given]
∴ ∠PAD ≅ ∠PCB … [Alternate angles theorem]
∠APD ≅ ∠CPB …[Vertically opposite angles]
∴ ∆APD ~ ∆CPB …[By AA test for similarity]
∴ \(\large \frac {AP}{PC}\) = \(\large \frac {PD}{BP}\)
i.e. \(\large \frac {AP}{PD}\) = \(\large \frac {PC}{BP}\) …[Alternendo]
Hence proved.
12. In fig 1.80, XY || seg AC. If 2AX = 3BX and XY = 9. Complete the activity to find the value of AC.
Activity :
2AX = 3BX
∴ \(\large \frac {AB}{BX}\) = \(\large \frac {□}{□}\)
∴ \(\large \frac {AX\,+\,BX}{BX}\) = \(\large \frac {□\,+\,□}{□}\) ………. by componendo.
∴ \(\large \frac {AB}{BX}\) = \(\large \frac {□}{□}\) ………. (I)
∴ ∆BCA ~ ∆BYX ………. □ test of similarity.
∴ \(\large \frac {BA}{BX}\) = \(\large \frac {AC}{XY}\) ………. corresponding sides of similar triangles.
∴ \(\large \frac {□}{□}\) = \(\large \frac {AC}{9}\) …from (I)
∴ AC = □ units
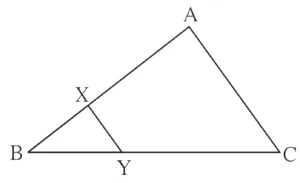
Solution:
2AX = 3BX
∴ \(\large \frac {AB}{BX}\) = \(\large \frac {3}{2}\)
∴ \(\large \frac {AX\,+\,BX}{BX}\) = \(\large \frac {3\,+\,2}{2}\) ………. by componendo.
∴ \(\large \frac {AB}{BX}\) = \(\large \frac {5}{2}\) ………. (I)
∴ ∆BCA ~ ∆BYX ………. AA test of similarity
∴ \(\large \frac {BA}{BX}\) = \(\large \frac {AC}{XY}\) ………. corresponding sides of similar triangles.
∴ \(\large \frac {5}{2}\) = \(\large \frac {AC}{9}\) …from (I)
∴ AC = 22.5 units
13. In figure 1.81, the vertices of square DEFG are on the sides of ∆ABC. ∠A = 90°. Then prove that DE² = BD × EC
(Hint : Show that ∆GBD is similar to ∆CFE. Use GD = FE = DE)
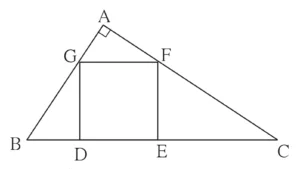
Given:
Vertices D, E, F, G are on the sides of ∆ABC
∠A = 90°
To Prove:
DE² = BD × EC
Hint:
Show that ∆GBD is similar to ∆CFE
Use GD = FE = DE
Solution:
□ DEFG is a square.
∴ DE = EF = GF = DG …(i) [All Sides of a square are equal]
seg GF || seg DE …[Opposite sides of a square are parallel]
∴ seg GF || side BC …[B – D – E – C]
Now,
seg GF || side BC and AB is the transversal
∠AGF ≅ ∠ABC …[Corresponding angles theorem]
∠AGF ≅ ∠GBD …(ii) [A – G – B, B – D – C]
Seg GF || side BC and AC is the transversal
∠AFG ≅ ∠ACB …[Corresponding angles theorem]
∠AFG ≅ ∠FCE …(iii) [A – F – C, C – E – B]
In ∆AGF and ∆DBG,
∠AGF ≅ ∠GBD …[From (ii)]
∠GAF ≅ ∠BDG …[Each is 90⁰]
∴ ∆AGF ~ ∆DBG …(iv) [By AA Test for similarity of triangles]
In ∆AGF and ∆EFC,
∠AFG ≅ ∠FCE …[From (iii)]
∠GAF ≅ ∠FEC …[Each is 90⁰]
∴ ∆AGF ~ ∆EFC …(v) [By AA Test for similarity of triangles]
∴ ∆DBG ~ ∆EFC …[From (iv) and (v)]
∴ \(\large \frac {BD}{EF}\) = \(\large \frac {DG}{EC}\) …[Corresponding sides are proportional]
∴ BD × EC = EF × DG
∴ BD × EC = DE × DE …[From (i)]
∴ DE² = BD × EC
Hence proved.
