Chapter 15 – Triangles and their Properties
Practice set 36
1. Observe the figures below and write the type of the triangle based on its angles.
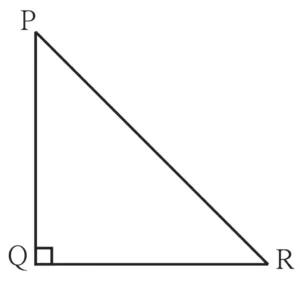
∆PQR is …… triangle.
Ans: right angled

∆XYZ is …… triangle.
Ans: obtuse angled
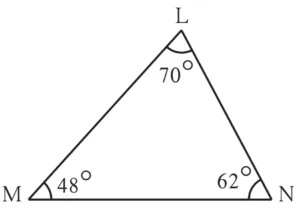
∆LMN is …… triangle.
Ans: acute angled
2. Observe the figures below and write the type of the triangle based on its sides.

∆ABC is ….. triangle
Ans: equilateral
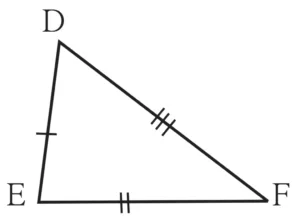
∆DEF is …… triangle.
Ans: scalene
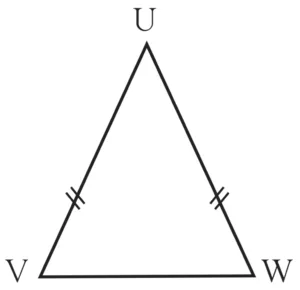
∆UVW is …… triangle.
Ans: isosceles
3. As shown in the figure, Avinash is standing near his house. He can choose from two roads to go to school. Which way is shorter? Explain why.
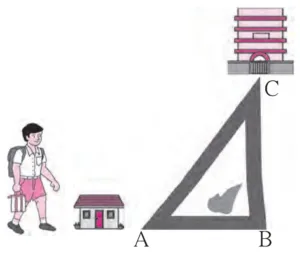
Ans: The two roads he can choose are (Road AB + Road BC) and Road AC. Avinash will choose AC road because by that road distance is less to school, so he will reach early to school. This AC distance is less because in a triangle sum of two sides is more than the third side.
i.e. AB + BC >AC
4. The lengths of the sides of some triangles are given. Say what types of triangles they are.
(1) 3 cm, 4 cm, 5 cm
Ans: No two sides have equal lengths, so the given triangle is a scalene triangle.
(2) 3.4 cm, 3.4 cm, 5 cm
Ans: Two sides have equal length, hence the given triangle is an isosceles triangle.
(3) 4.3 cm, 4.3 cm, 4.3 cm
Ans: All the three sides have equal lengths, therefore, the given triangle is an equilateral triangle.
(4) 3.7 cm, 3.4 cm, 4 cm
Ans: Since, no two sides have equal lengths, the given triangle is a scalene triangle.
5. The lengths of three segments are given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
(1) 17 cm, 7 cm, 8 cm
Ans: The lengths of the three sides are 17 cm, 7 cm, 8 cm.
(i) 7 cm + 17 cm = 24 cm
Greater than 8 cm
(ii) 8 cm +17 cm = 25 cm
Greater than 7 cm
(iii) 7 cm + 8 cm = 15 cm
Smaller than 17 cm
The sum of lengths of two sides is not greater than the length of the third side.
∴ The triangle cannot be drawn with sides 17 cm, 7 cm, 8 cm.
(2) 7 cm, 24 cm, 25 cm
Ans: The lengths of the three sides are 7 cm, 24 cm, 25 cm.
(i) 7 cm + 24 cm = 31 cm,
Greater than 25 cm
(ii) 25 cm + 7 cm = 32 cm
Greater than 24 cm
(iii) 24 cm + 25 cm = 49 cm
Greater than 7 cm
The sum of lengths of two sides is greater than the length of the third side.
∴ The triangle can be drawn with sides 7 cm, 24 cm, 25 cm.
(3) 9 cm, 6 cm, 16 cm
Ans: The lengths of the three sides are 9 cm, 6 cm, 16 cm.
(i) 9 cm + 16 cm = 25 cm
Greater than 6 cm
(ii) 6 cm + 16 cm = 22 cm
Greater than 9 cm
(iii) 9 cm+ 6 cm =15 cm
Smaller than 16 cm
The sum of lengths of two sides is not greater than the length of the third side.
∴ The triangle cannot be drawn with sides 9 cm, 6 cm, 16 cm.
(4) 8.4 cm, 16.4 cm, 4.9 cm
Ans: The lengths of the three sides are 8.4 cm, 16.4 cm, 4.9 cm.
(i) 8.4 cm + 16.4 cm = 24.8 cm
Greater than 4.9 cm
(ii) 4.9 cm + 16.4 cm = 21.3 cm
Greater than 8.4 cm
(iii) 8.4 cm + 4.9 cm = 13.3 cm
Smaller than 16.4 cm
The sum of lengths of two sides is not greater than the length of the third side.
∴ The triangle cannot be drawn with sides 8.4 cm, 16.4 cm, 4.9 cm.
(5) 15 cm, 20 cm, 25 cm
Ans: The lengths of the three sides are 15 cm, 20 cm, 25 cm.
(i) 15 cm + 20 cm = 35 cm
Greater than 25 cm
(ii) 25 cm + 20 cm = 45 cm
Greater than 15 cm
(iii) 15 cm + 25 cm = 40 cm
Greater than 20 cm
The sum of lengths of two sides is greater than the length of the third side.
∴ The triangle can be drawn with sides 15 cm, 20 cm, 25 cm.
(6) 12 cm, 12 cm, 16 cm
Ans: The lengths of the three sides are 12 cm, 12 cm, 16 cm.
(i) 12 cm + 12 cm = 24 cm
Greater than 16 cm
(ii) 12 cm + 16 cm = 28 cm
Greater than 12 cm
(iii)12 cm + 16 cm = 28 cm
Greater than 12 cm
The sum of lengths of two sides is greater than the length of the third side.
∴ The triangle can be drawn with sides 12 cm, 12 cm, 16 cm.
