Chapter 12 - Perimeter and Area
Problem Set 48
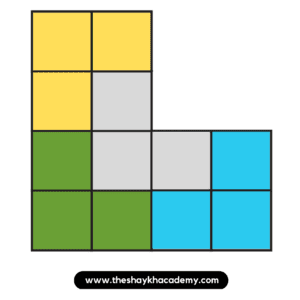
1. Write the perimeter of each figure in the box given below it.
(i)

Solution:
Perimeter of any figure = Sum of lengths of all sides
∴ Perimeter of given figure = 20 + 16 + 7 + 14
∴ Perimeter of given figure = 57 cm
Ans: Perimeter of given figure = 57 cm
(ii)
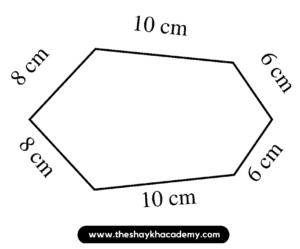
Solution:
Perimeter of any figure = Sum of lengths of all sides
∴ Perimeter of given figure = 10 + 6 + 6 + 10 + 8 + 8
∴ Perimeter of given figure = 48 cm
Ans: Perimeter of given figure = 48 cm
(iii)
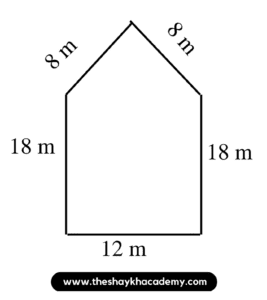
Solution:
Perimeter of any figure = Sum of lengths of all sides
∴ Perimeter of given figure = 12 + 18 + 8 + 8 + 18
∴ Perimeter of given figure = 64 cm
Ans: Perimeter of given figure = 64 cm
2. If a square of side 1 cm is cut out of the corner of a larger square with side 3 cm (see the figure), what will be the perimeter of the remaining shape?
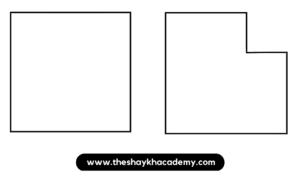
Solution:
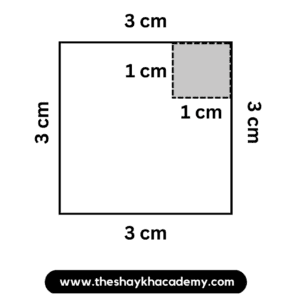

Perimeter of the remaining shape = 2 + 3 + 3 + 2 + 1 + 1
∴ Perimeter of the remaining shape = 12 cm
Ans: Perimeter of the remaining shape will be 12 cm.
Problem Set 49
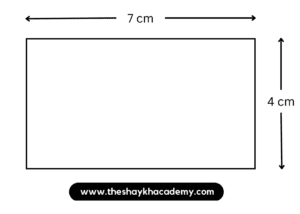
1. How much wire will be needed to make a rectangle 7 cm long and 4 cm wide?
Solution:
Perimeter of a rectangle = 2 × length + 2 × breadth
∴ Perimeter of the rectangle = 2 × 7 + 2 × 4
∴ Perimeter of the rectangle = 14 + 8
∴ Perimeter of the rectangle = 22 cm
Ans: 22 cm wire will be needed to make a rectangle.
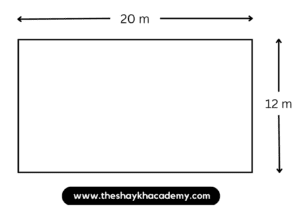
2. If the length of a rectangle is 20m and its width is 12m, what is its perimeter?
Solution:
Perimeter of a rectangle = 2 × length + 2 × breadth
∴ Perimeter of the rectangle = 2 × 20 + 2 × 12
∴ Perimeter of the rectangle = 40 + 24
∴ Perimeter of the rectangle = 64 cm
Ans: Perimeter of the rectangle is 64 cm.
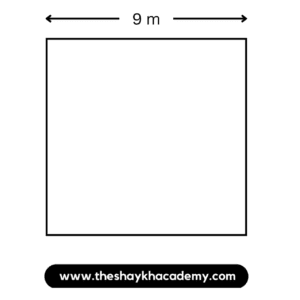
3. Each side of a square is 9m long. Find its perimeter.
Solution:
Perimeter of a square = 4 × length of one side
∴ Perimeter of the square = 4 × 9
∴ Perimeter of the square = 36 m
Ans: Perimeter of the square is 36 m.
4. If we take 4 rounds around a field that is 160 m long and 90 m wide, what is the distance we walk in kilometres?

Solution:
Perimeter of a rectangle = 2 × length + 2 × breadth
∴ Perimeter of the rectangular field = 2 × 160 + 2 × 90
∴ Perimeter of the rectangle = 320 + 180
∴ Perimeter of the rectangle = 500 cm
Distance walked in 1 round is 500 m.
Distance walked in 4 rounds is ?
∴ Distance walked in 4 rounds = 500 × 4
∴ Distance walked in 4 rounds = 2000 m
Now,
1000 m = 1 km
∴ 2000 m = 2 × 1000 m = 2 km
∴ Distance walked in 4 rounds = 2 km
Ans: The distance walked in 4 rounds is 2 km
5. Sanju completes 12 rounds around a square park every day. If one side of the park is 120 m, find out in kilometres and metres the distance that Sanju covers daily.
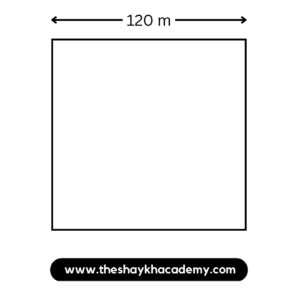
Solution:
Perimeter of a square = 4 × length of one side
∴ Perimeter of the square park = 4 × 120
∴ Perimeter of the rectangle = 480 m
Distance walked in 1 round is 480 m.
Distance walked in 12 rounds is ?
∴ Distance walked in 12 rounds = 480 × 12
∴ Distance walked in 12 rounds = 5760 m
Now,
1000 m = 1 km
∴ 5760 m = 5 × 1000 m + 760 m = 5 km 760 m
∴ Distance walked in 12 rounds (in m) = 5760 m and (in km) = 5 km 760 m
Ans: Sanju covers 5 km 760 m or 5760 m daily.
Solution:
Perimeter of a square = 4 × length of one side
∴ Perimeter of the square park = 4 × 120
∴ Perimeter of the rectangle = 480 m
Distance walked in 1 round is 480 m.
Distance walked in 12 rounds is ?
∴ Distance walked in 12 rounds = 480 × 12
∴ Distance walked in 12 rounds = 5760 m
Now,
1000 m = 1 km
∴ 5760 m = 5 × 1000 m + 760 m = 5 km 760 m
∴ Distance walked in 12 rounds (in m) = 5760 m and (in km) = 5 km 760 m
Ans: Sanju covers 5 km 760 m or 5760 m daily.
6. The length of a rectangular plot of land is 50 m and its width is 30 m. A triple fence has to be put along its edges. If the wire costs 60 rupees per metre, what will be the total cost of the wire needed for the fence?
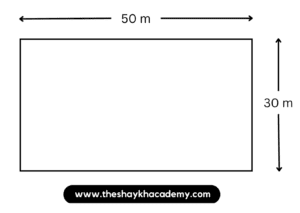
Solution:
Perimeter of a rectangle = 2 × length + 2 × breadth
Perimeter of the rectangular plot = 2 × 50 + 2 × 30
∴ Perimeter of the rectangular plot = 100 + 60
∴ Perimeter of the rectangular plot = 160 m
Wire needed for a triple fence = 3 × 160
∴ Wire needed for a triple fence = 480 m
Cost of the wire needed = Wire needed × rate of the wire
∴ Cost of the wire needed = 480 × 60
∴ Cost of the wire needed = ₹ 28,800
Ans: The total cost of the wire needed for the fence is ₹ 28,800.
7. A game requires its players to run around a square playground. Each side of the playground is 20 m long. One player took 5 rounds around the playground. How many metres did he run altogether?

Solution:
Perimeter of a square = 4 × length of one side
∴ Perimeter of the square playground = 4 × 20
∴ Perimeter of the square playground = 80 m
Distance run in 1 round is 80 m.
Distance run in 5 rounds is ?
∴ Distance run in 5 rounds = 80 × 5
∴ Distance run in 5 rounds = 400 m
Ans: The player runs 400 m altogether.
8. Four rounds of wire fence have to be put around a field. If the field is 60 m long and 40 m wide, how much wire will be needed?
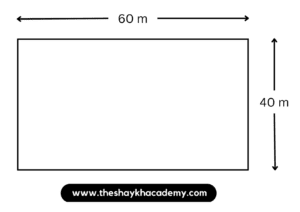
Solution:
Perimeter of a rectangle = 2 × length + 2 × breadth
Perimeter of the rectangular field = 2 × 60 + 2 × 40
∴ Perimeter of the rectangular field = 120 + 80
∴ Perimeter of the rectangular field = 200 m
Wire required for 1 round is 200 m
∴ Wire required for 4 rounds is ?
∴ Wire required for 4 rounds = 200 × 4
∴ Wire required for 4 rounds = 800 m
Ans: Wire required for 4 rounds is 800 m.
9. The sides of a triangle are 24.7cm, 20.4 cm and 10.5 cm respectively. What is the perimeter of the triangle?
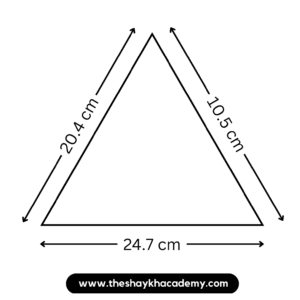
Solution:
Perimeter of any figure = Sum of lengths of all sides
∴ Perimeter of the triangle = 24.7 + 20.4 + 10.5
∴ Perimeter of the triangle = 55.6 m
Ans: The perimeter of a triangle is 55.6 cm.
10. Look at the figures on the sheet of graph paper. Measure their sides with the help of the lines on the graph paper. Write the perimeter of each in the right box

(1) Perimeter of rectangle ABCD = ___ cm
Solution:
From figure,
AB = 3.5 m
BC = 2.5 m
∴ Perimeter of rectangle ABCD = 2 × 3.5 + 2 × 2.5
∴ Perimeter of rectangle ABCD = 7 + 5
∴ Perimeter of rectangle ABCD = 12 m
Ans: Perimeter of rectangle ABCD = 12 cm
(2) Perimeter of rectangle EFGH = ___ cm
Solution:
From figure,
EF = 3.8 m
FG = 1.3 m
∴ Perimeter of rectangle EFGH = 2 × 3.8 + 2 × 1.3
∴ Perimeter of rectangle EFGH = 7.6 + 2.6
∴ Perimeter of rectangle EFGH = 10.2 m
Ans: Perimeter of rectangle EFGH = 10.2 cm
(3) Perimeter of square PQRS = ___ cm
Solution:
From figure,
PQ = 2.4 m
∴ Perimeter of square PQRS = 4 × 2.4
∴ Perimeter of square PQRS = 9.6 m
Ans: Perimeter of rectangle EFGH = 10.2 cm
(4) Perimeter of rectangle STUV = ___ cm
Solution:
From figure,
SV = 3 m
VU = 2 m
∴ Perimeter of rectangle STUV = 2 × 3 + 2 × 2
∴ Perimeter of rectangle STUV = 6 + 4
∴ Perimeter of rectangle STUV = 10 m
Ans: Perimeter of rectangle STUV = 10 cm.
Problem Set 50
1. The length of the side of each square is given below. Find its area.
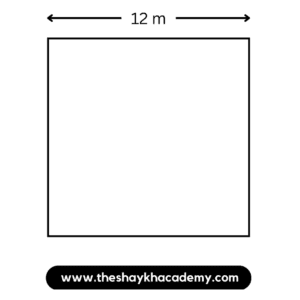
(1) 12 metres
Solution:
Side of the square = 12 metres
Area of a square = side × side
∴ Area of the square = 12 × 12
∴ Area of the square = 144 sq.m
Ans: Area of the square = 144 sq.m
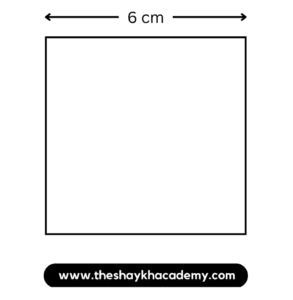
(2) 6 cm
Solution:
Side of the square = 6 cm
Area of a square = side × side
∴ Area of the square = 6 × 6
∴ Area of the square = 36 sq.cm
Ans: Area of the square = 36 sq.cm
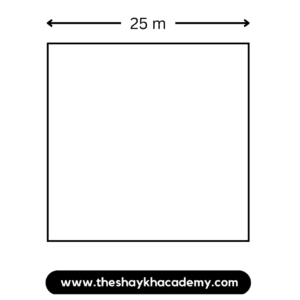
(3) 25 metres
Solution:
Side of the square = 25 metres
Area of a square = side × side
∴ Area of the square = 25 × 25
∴ Area of the square = 625 sq.m
Ans: Area of the square = 625 sq.m
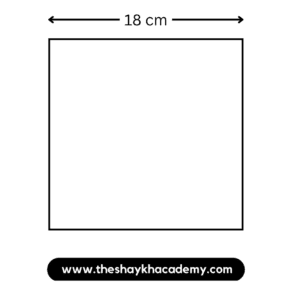
(4) 18 cm
Solution:
Side of the square = 18 cm
Area of a square = side × side
∴ Area of the square = 18 × 18
∴ Area of the square = 324 sq.cm
Ans: Area of the square = 324 sq.cm
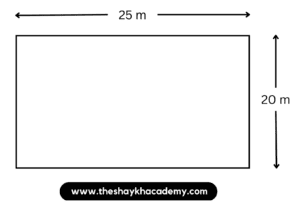
2. If the cost of 1 sq m of a plot of land is 900 rupees, find the total cost of a plot of land that is 25 m long and 20 m broad.
Solution:
Length of plot = 25 m
Breadth of plot = 20 m
Area of a rectangle = length × breadth
∴ Area of the rectangular plot = 25 × 20
∴ Area of the rectangular plot = 500 sq.m.
Cost of 1 sq.m of plot is ₹ 900
∴ Cost of 500 sq.m of plot is ?
Cost of 500 sq.m of plot = Area of the plot × rate
∴ Cost of 500 sq.m of plot = 500 × 900
∴ Cost of 500 sq.m of plot = ₹ 4,50,000
Ans: Cost of 500 sq.m of plot is ₹ 4,50,000
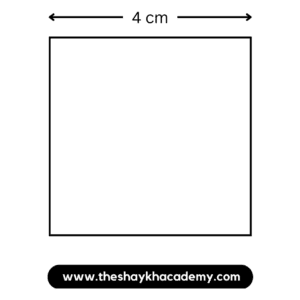
3. The side of a square is 4 cm. The length of a rectangle is 8 cm and its width is 2 cm. Find the perimeter and area of both figures.
Solution:
Side of a square = 4 cm
Perimeter of a square = 4 × side
∴ Perimeter of the square = 4 × 4
∴ Perimeter of the square = 16 cm
Area of a square = side × side
∴ Area of the square = 4 × 4
∴ Area of the square = 16 sq.cm
Length of a rectangle = 8 cm
Breadth of a rectangle = 2 cm
Perimeter of a rectangle = 2 × length + 2 × breadth
∴ Perimeter of the rectangle = 2 × 8 + 2 × 2
∴ Perimeter of the rectangle = 16 + 4
∴ Perimeter of the rectangle = 20 cm
Area of a rectangle = length × breadth
∴ Area of a rectangle = 8 × 2
∴ Area of a rectangle = 16 sq.cm
Ans: Perimeter of the square is 16 cm and its area is 16 sq.cm and perimeter of the rectangle is 20 cm and its area is 16 sq.cm
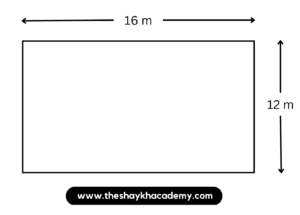
4. What will be the labour cost of laying the floor of an assembly hall that is 16 m long and 12 m wide if the cost of laying 1 sq m is 80 rupees ?
Solution:
Length of plot = 16 m
Breadth of plot = 12 m
Area of a rectangle = length × breadth
∴ Area of the rectangular plot = 16 × 12
∴ Area of the rectangular plot = 192 sq.m.
Cost of laying 1 sq.m is ₹ 80
∴ Cost of laying 192 sq.m. is ?
∴ Cost of laying 192 sq.m. = 192 × 80
∴ Cost of laying 192 sq.m. = ₹ 15,360
Ans: The labour cost of laying the floor of the assembly hall is ₹ 15,360
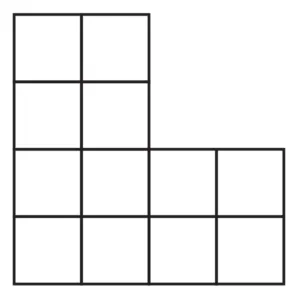
5. The picture alongside shows some squares. Find out how many squares with the same measures will fit in the empty space in the figure.
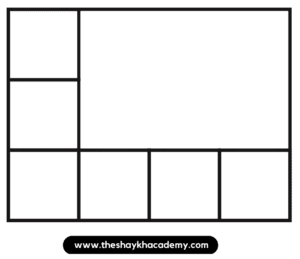
Solution:
Length of the empty space = 4 – 1
∴ Length of the empty space = 3 cm
Breadth of the empty space = 3 – 1
∴ Breadth of the empty space = 2 cm
To find the no of squares in empty space, we should find area of the empty space.
Area of the empty space = length x breadth
∴ Area of the empty space = 3 x 2
∴ Area of the empty space = 6 sq.cm
Ans: 6 squares will fit in the empty space in the figure.
6. Divide the figure given alongside into four parts in such a way that the area and shape of each part is the same. Colour the parts with different colours.
Solution: